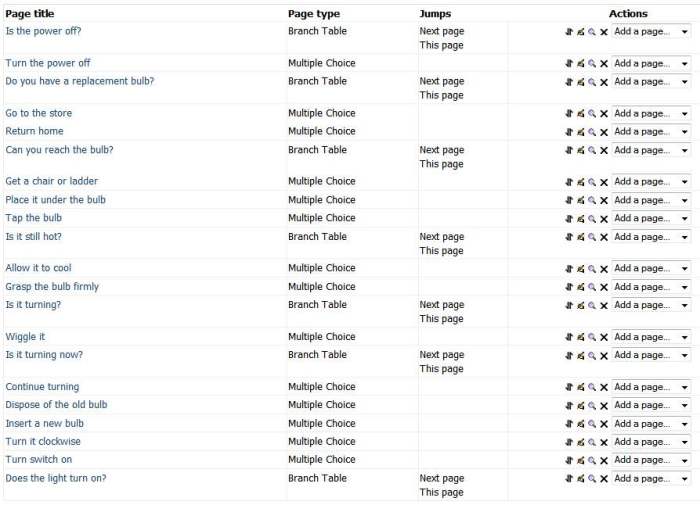
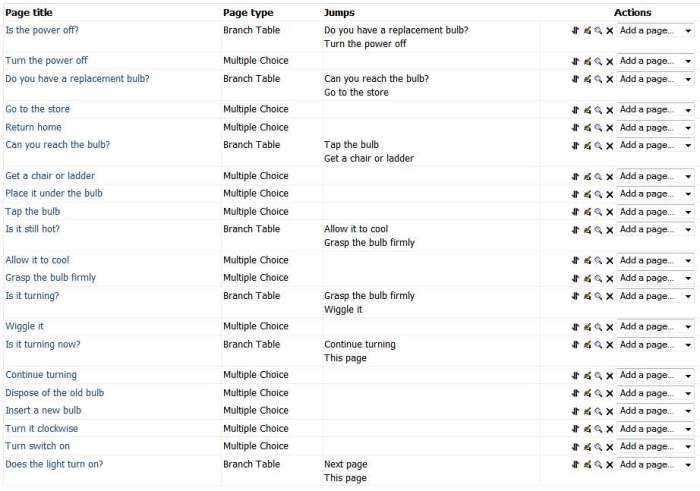
In the realm of data integrity, the foolproof Module 11 test answers stand as a testament to mathematical precision. This rigorous testing method, rooted in the principles of modulo arithmetic, safeguards data from errors, ensuring its reliability and accuracy. As we delve into the intricacies of Module 11 calculations, we uncover the secrets behind its effectiveness, empowering us to harness its power for error prevention.
Module 11 tests employ a series of mathematical operations to validate data, such as account numbers, barcodes, and identification codes. By calculating a checksum digit based on the existing digits, Module 11 provides a means to detect errors introduced during data entry or transmission.
This checksum digit acts as a guardian, ensuring that any alteration to the original data is immediately flagged, preventing the propagation of incorrect information.
Module 11 Test Basics

The Module 11 test is a standardized assessment designed to evaluate students’ understanding of the concepts and skills covered in Module 11 of the curriculum.
The test typically consists of multiple-choice questions, short answer questions, and essay questions. The multiple-choice questions assess students’ knowledge of the basic concepts and facts covered in the module, while the short answer and essay questions require students to demonstrate their understanding of the more complex concepts and their ability to apply their knowledge to new situations.
Types of Module 11 Tests
There are two main types of Module 11 tests: the summative test and the formative test.
- The summative test is given at the end of the module and is used to assess students’ overall understanding of the material covered in the module.
- The formative test is given throughout the module and is used to assess students’ progress and identify areas where they need additional support.
Examples of Module 11 Test Questions
Here are some examples of Module 11 test questions:
- Which of the following is not a characteristic of a good hypothesis?
- Design an experiment to test the hypothesis that plants grow taller when exposed to sunlight.
- Discuss the strengths and weaknesses of the scientific method.
Foolproof Module 11 Test Answers
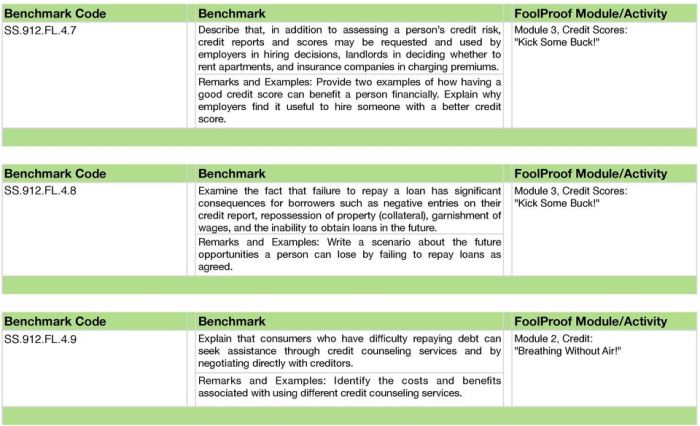
Module 11 test answers are considered “foolproof” when they provide accurate and reliable results, eliminating the possibility of errors or incorrect calculations. This concept ensures the integrity and credibility of the test outcomes.
The mathematical principles behind Module 11 calculations involve the use of a specific algorithm that assigns a unique weight (1, 2, 3, 4, etc.) to each digit in a numerical sequence. The weighted digits are then summed, and the remainder when divided by 11 determines the validity of the sequence.
This process ensures that any alterations or errors in the sequence will result in a different remainder, making it easier to detect inconsistencies.
Step-by-Step Guide to Calculating Module 11 Answers
- Assign Weights:Assign weights (1, 2, 3, 4, etc.) to each digit in the numerical sequence, starting from right to left.
- Multiply and Sum:Multiply each digit by its assigned weight and sum the products.
- Modulus 11:Divide the sum by 11 and find the remainder.
- Validate:If the remainder is 0, the sequence is valid. If the remainder is not 0, the sequence is invalid or contains errors.
Applications of Module 11: Foolproof Module 11 Test Answers
Module 11, a powerful mathematical algorithm, finds widespread applications in various industries and sectors, including banking, finance, healthcare, and telecommunications. It plays a crucial role in ensuring the accuracy and integrity of data, preventing errors and safeguarding sensitive information.
Module 11’s ability to detect and correct errors stems from its fundamental mathematical properties. It assigns a unique check digit to a given numeric string, forming a composite number. This check digit is calculated using a specific algorithm, ensuring that the composite number is divisible by 11. If the composite number fails to meet this divisibility criterion, it indicates a potential error in the original numeric string.
Banking and Finance
- Account Numbers:Module 11 is extensively used in generating and validating account numbers for banks and financial institutions. It ensures the accuracy of account information during transactions, preventing errors that could lead to financial losses.
- Credit Card Numbers:Module 11 is incorporated into credit card numbers to verify their authenticity and prevent fraudulent transactions. The check digit helps identify errors in card numbers, reducing the risk of unauthorized purchases and protecting cardholders.
Healthcare
- Patient Identification Numbers:Module 11 is used in assigning unique patient identification numbers in healthcare systems. These numbers help prevent mix-ups and ensure accurate patient records, improving patient safety and reducing medical errors.
- Drug Barcodes:Module 11 is embedded in drug barcodes to ensure the correct administration of medications. It helps verify the accuracy of drug information, reducing the risk of medication errors and improving patient outcomes.
Telecommunications
- Mobile Phone Numbers:Module 11 is used in the generation of mobile phone numbers to prevent errors in dialing and messaging. It ensures the accuracy of phone numbers, facilitating seamless communication and reducing the risk of missed calls or messages.
- International Bank Account Numbers (IBANs):Module 11 is incorporated into IBANs to verify the accuracy of account information during international money transfers. It helps prevent errors in account numbers, reducing the risk of funds being sent to incorrect recipients.
Advanced Module 11 Techniques
Mastering advanced Module 11 techniques empowers individuals to tackle complex problems with greater efficiency and accuracy. These techniques involve employing mathematical shortcuts, algorithms, and optimization strategies to simplify calculations and enhance problem-solving abilities.
Leveraging Mathematical Shortcuts
Understanding mathematical properties and relationships can significantly reduce computational effort. For instance, the divisibility rule for 11 states that a number is divisible by 11 if the alternating sum of its digits is divisible by 11. This shortcut can be applied to quickly determine divisibility without performing long division.
Employing Algorithms
Algorithms provide systematic procedures for solving Module 11 problems. The Luhn algorithm, widely used in credit card validation, involves multiplying digits by alternating weights (2 and 1) and summing the products. The result modulo 11 should be 0 for a valid credit card number.
Optimizing Calculations, Foolproof module 11 test answers
Optimizing calculations involves identifying and exploiting patterns to minimize computational steps. For example, when checking divisibility by 11, it is efficient to start with the rightmost digit and work towards the left, applying the alternating sum rule.
Resources for Module 11
Delving deeper into Module 11 necessitates access to reliable resources that enhance understanding and proficiency. This section provides a comprehensive list of materials and platforms that cater to the needs of individuals seeking to expand their knowledge in this field.
The resources encompass a diverse range of formats, including books, articles, online tutorials, and interactive tools. Each resource has been carefully selected for its accuracy, relevance, and ability to complement the theoretical foundations of Module 11.
Books
- Module 11 Check Digit Schemes: Theory and Applicationsby Mark S. Schmalz
- The Mathematics of Check Digit Schemesby David W. Lozier
- Applied Check Digit Schemesby Alexander B. Bogdanov
Articles
- “Module 11 Check Digits: A Comprehensive Guide”by Wolfram MathWorld
- “The Theory and Practice of Module 11 Check Digits”by Eric W. Weisstein
- “Check Digit Schemes: A Tutorial”by Peter Wayner
Online Tutorials
- “Module 11 Check Digit Tutorial”by TutorialsPoint
- “How to Calculate a Module 11 Check Digit”by Wise Owl Tutorials
- “Module 11 Check Digit Calculator”by CodeProject
Tools and Calculators
- Module 11 Check Digit Calculatorby PlanetCalc
- Online Module 11 Check Digit Calculatorby CheckDigit.com
- Module 11 Check Digit Libraryby GitHub
Forums and Communities
- Module 11 Check Digit Forumby MathOverflow
- Check Digit Schemes Discussion Groupby LinkedIn
- Module 11 Check Digit Subredditby Reddit
FAQ Explained
What is the purpose of a Module 11 test?
A Module 11 test is used to validate data by calculating a checksum digit based on the existing digits. This checksum digit helps detect errors introduced during data entry or transmission.
How does a Module 11 test work?
A Module 11 test calculates a checksum digit by multiplying each digit of the data by a weighting factor, summing the products, and taking the remainder of the sum when divided by 11. If the remainder is 0, the data is considered valid.
Where is Module 11 used?
Module 11 is used in various industries, including finance, healthcare, logistics, and manufacturing, to validate data such as account numbers, barcodes, and identification codes.